


Next: Hyperfine Structure
Up: Time-Independent Perturbation Theory
Previous: Fine Structure of Hydrogen
Consider a hydrogen atom placed in a uniform 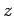 -directed external
magnetic field of strength
-directed external
magnetic field of strength  . The modification to the Hamiltonian
of the system is
. The modification to the Hamiltonian
of the system is
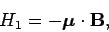 |
(992) |
where
 |
(993) |
is the total electron magnetic moment, including both orbital and spin contributions
[see Eqs. (758)-(760)]. Thus,
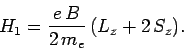 |
(994) |
Suppose that the applied magnetic field is much weaker than the atom's internal
magnetic field (977). Since the magnitude of the internal
field is about 25 tesla, this is a fairly reasonable assumption. In this
situation, we can treat 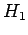 as a small perturbation acting
on the simultaneous eigenstates of the unperturbed Hamiltonian and
the fine structure Hamiltonian. Of course, these states
are the simultaneous eigenstates of
as a small perturbation acting
on the simultaneous eigenstates of the unperturbed Hamiltonian and
the fine structure Hamiltonian. Of course, these states
are the simultaneous eigenstates of  ,
, 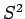 ,
, 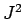 , and
, and 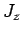 (see
previous section). Hence, from standard perturbation theory, the
first-order energy-shift induced by a weak external magnetic field
is
(see
previous section). Hence, from standard perturbation theory, the
first-order energy-shift induced by a weak external magnetic field
is
since 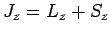 . Now, according to Eqs. (825) and
(826),
. Now, according to Eqs. (825) and
(826),
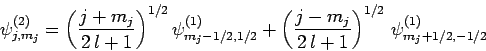 |
(996) |
when 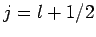 , and
, and
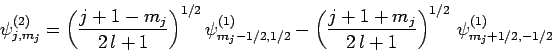 |
(997) |
when 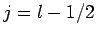 . Here, the
. Here, the
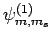 are the
simultaneous eigenstates of
are the
simultaneous eigenstates of  ,
, 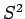 ,
,  , and
, and  , whereas
the
, whereas
the
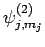 are the simultaneous eigenstates of
are the simultaneous eigenstates of
 ,
, 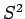 ,
, 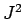 , and
, and 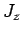 . In particular,
. In particular,
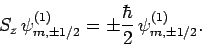 |
(998) |
It follows from Eqs. (996)-(998), and the
orthormality of the 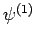 , that
, that
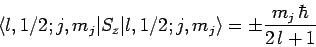 |
(999) |
when 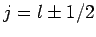 . Thus, the induced energy-shift when a hydrogen atom is placed in an external magnetic field--which is known as the Zeeman effect--becomes
. Thus, the induced energy-shift when a hydrogen atom is placed in an external magnetic field--which is known as the Zeeman effect--becomes
![\begin{displaymath}
\Delta E_{l,1/2;j,m_j} = \mu_B B m_j\left[1\pm \frac{1}{2 l+1}\right]
\end{displaymath}](img2348.png) |
(1000) |
where the 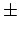 signs correspond to
signs correspond to 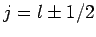 . Here,
. Here,
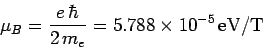 |
(1001) |
is known as the Bohr magnetron. Of course, the quantum number  takes values differing by unity in the range
takes values differing by unity in the range 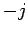 to
to 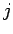 . It, thus,
follows from Eq. (1000) that the Zeeman effect splits
degenerate states characterized by
. It, thus,
follows from Eq. (1000) that the Zeeman effect splits
degenerate states characterized by 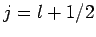 into
into  equally spaced states of interstate spacing
equally spaced states of interstate spacing
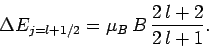 |
(1002) |
Likewise, the Zeeman effect splits degenerate states characterized by
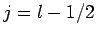 into
into  equally spaced states of interstate spacing
equally spaced states of interstate spacing
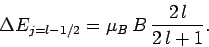 |
(1003) |
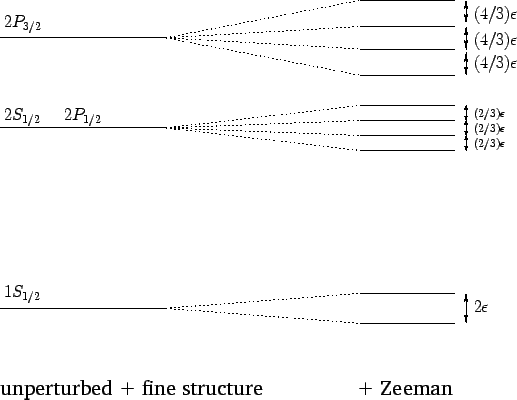
In conclusion, in the presence of a weak external magnetic field, the two degenerate 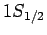 states of the hydrogen atom are split by
states of the hydrogen atom are split by  . Likewise,
the four degenerate
. Likewise,
the four degenerate 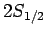 and
and  states are split by
states are split by
 , whereas the four degenerate
, whereas the four degenerate 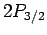 states
are split by
states
are split by
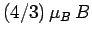 . This is illustrated in Fig. 24.
Note, finally, that since the
. This is illustrated in Fig. 24.
Note, finally, that since the
 are not simultaneous
eigenstates of the unperturbed and perturbing Hamiltonians,
Eqs. (1002) and (1003) can only be regarded as the expectation
values of the magnetic-field induced energy-shifts. However, as long as
the external magnetic field is much weaker than the internal magnetic
field, these expectation values are almost identical to the actual
measured values of the energy-shifts.
are not simultaneous
eigenstates of the unperturbed and perturbing Hamiltonians,
Eqs. (1002) and (1003) can only be regarded as the expectation
values of the magnetic-field induced energy-shifts. However, as long as
the external magnetic field is much weaker than the internal magnetic
field, these expectation values are almost identical to the actual
measured values of the energy-shifts.
Figure 24:
The Zeeman effect for the
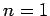 and
and 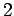 states of a hydrogen atom. Here,
states of a hydrogen atom. Here,
 . Not to scale.
. Not to scale.
 |



Next: Hyperfine Structure
Up: Time-Independent Perturbation Theory
Previous: Fine Structure of Hydrogen
Richard Fitzpatrick
2010-07-20
![]() as a small perturbation acting
on the simultaneous eigenstates of the unperturbed Hamiltonian and
the fine structure Hamiltonian. Of course, these states
are the simultaneous eigenstates of
as a small perturbation acting
on the simultaneous eigenstates of the unperturbed Hamiltonian and
the fine structure Hamiltonian. Of course, these states
are the simultaneous eigenstates of ![]() ,
, ![]() ,
, ![]() , and
, and ![]() (see
previous section). Hence, from standard perturbation theory, the
first-order energy-shift induced by a weak external magnetic field
is
(see
previous section). Hence, from standard perturbation theory, the
first-order energy-shift induced by a weak external magnetic field
is
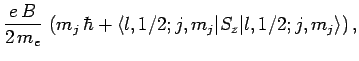
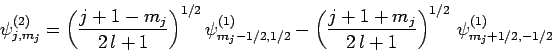
![]() states of the hydrogen atom are split by
states of the hydrogen atom are split by ![]() . Likewise,
the four degenerate
. Likewise,
the four degenerate ![]() and
and ![]() states are split by
states are split by
![]() , whereas the four degenerate
, whereas the four degenerate ![]() states
are split by
states
are split by
![]() . This is illustrated in Fig. 24.
Note, finally, that since the
. This is illustrated in Fig. 24.
Note, finally, that since the
![]() are not simultaneous
eigenstates of the unperturbed and perturbing Hamiltonians,
Eqs. (1002) and (1003) can only be regarded as the expectation
values of the magnetic-field induced energy-shifts. However, as long as
the external magnetic field is much weaker than the internal magnetic
field, these expectation values are almost identical to the actual
measured values of the energy-shifts.
are not simultaneous
eigenstates of the unperturbed and perturbing Hamiltonians,
Eqs. (1002) and (1003) can only be regarded as the expectation
values of the magnetic-field induced energy-shifts. However, as long as
the external magnetic field is much weaker than the internal magnetic
field, these expectation values are almost identical to the actual
measured values of the energy-shifts.