


Next: Exercises
Up: Wave-Particle Duality
Previous: Schrödinger's Equation
Collapse of the Wave Function
Consider an extended wavefunction 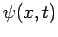 . According to our
usual interpretation,
. According to our
usual interpretation,
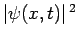 is proportional to the
probability density of a measurement of the particle's position yielding the
value
is proportional to the
probability density of a measurement of the particle's position yielding the
value  at time
at time  . If the wavefunction is extended then there is a wide
range of likely values that this measurement could give.
Suppose that we make such a measurement, and obtain the value
. If the wavefunction is extended then there is a wide
range of likely values that this measurement could give.
Suppose that we make such a measurement, and obtain the value 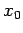 .
We now know that the particle is located at
.
We now know that the particle is located at 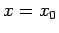 .
If we make another measurement immediately after the first one then
what value do we expect to obtain? Well, common sense tells us that
we must obtain the same value,
.
If we make another measurement immediately after the first one then
what value do we expect to obtain? Well, common sense tells us that
we must obtain the same value, 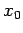 , since the particle
cannot have shifted position appreciably in an infinitesimal time interval.
Thus, immediately after the first measurement, a measurement of
the particle's position is certain to give the value
, since the particle
cannot have shifted position appreciably in an infinitesimal time interval.
Thus, immediately after the first measurement, a measurement of
the particle's position is certain to give the value 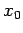 , and has
no chance of giving any other value. This implies that the
wavefunction must have collapsed to some sort of ``spike'' function
located at
, and has
no chance of giving any other value. This implies that the
wavefunction must have collapsed to some sort of ``spike'' function
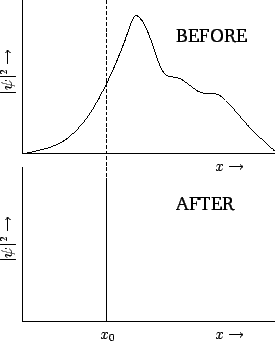
located at 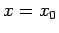 . This is illustrated in Fig. 9.
Of course, as soon as the wavefunction has collapsed, it starts to
expand again, as discussed in Sect. 3.13. Thus, the second measurement
must be made reasonably quickly after the first, in order to guarantee that the
same result will be obtained.
. This is illustrated in Fig. 9.
Of course, as soon as the wavefunction has collapsed, it starts to
expand again, as discussed in Sect. 3.13. Thus, the second measurement
must be made reasonably quickly after the first, in order to guarantee that the
same result will be obtained.
Figure 9:
Collapse of the wavefunction upon measurement of  .
.
 |
The above discussion illustrates an important point in quantum
mechanics. Namely, that the wavefunction of a particle
changes discontinuously (in time) whenever a measurement is made. We conclude that there are two types of time
evolution of the wavefunction in quantum mechanics. First, there is a smooth evolution which is governed
by Schrödinger's equation. This evolution takes place between measurements. Second, there is a discontinuous evolution which
takes place each time a measurement is made.
Subsections



Next: Exercises
Up: Wave-Particle Duality
Previous: Schrödinger's Equation
Richard Fitzpatrick
2010-07-20
